

Let us take an arithmetic sequence that has its first term to be a 1 and the common difference to be d. We can use this formula to be more helpful for larger values of 'n'. Substituting these values in the sum sum of arithmetic sequence formula,

We have to calculate her earnings in the first 5 years. Natalie for the first year is, a = 2,00,000. Then how much does she earn at the end of the first 5 years? Natalie earns $200,000 per annum and her salary increases by $25,000 per annum. When the n th term is known: S n = n/2.When the n th term is NOT known: S n= n/2.The sum of its first n terms is denoted by S n.
#Explicit formula for arithmetic sequence guided notes series#
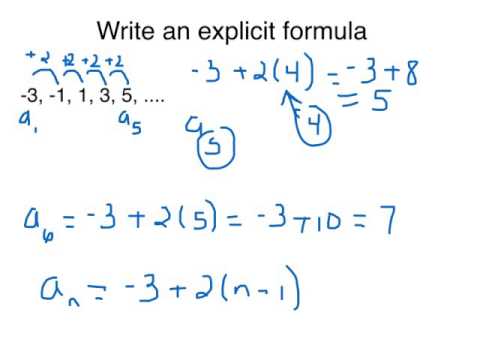
Consider an arithmetic series in which the first term is a 1 (or 'a') and the common difference is d. Note that the sum of terms of an arithmetic sequence is known as arithmetic series. The sum of the arithmetic sequence formula is used to find the sum of its first n terms. This formula just follows the definition of the arithmetic sequence.Įxample: Find a 21 of an arithmetic sequence if a 19 = -72 and d = 7. There is another formula to find the n th term which is called the " recursive formula of an arithmetic sequence" and is used to find a term (a n) of the sequence when its previous term (a n-1) and 'd' are known. The above formula for finding the n t h term of an arithmetic sequence is used to find any term of the sequence when the values of 'a 1' and 'd' are known. The following table shows some arithmetic sequences along with the first term, the common difference, and the n th term. This directly follows from the understanding that the arithmetic sequence a 1, a 2, a 3. This is also known as the general term of the arithmetic sequence. The n th term of an arithmetic sequence a 1, a 2, a 3. = 3, 6, 9, 12,15.Ī few more examples of an arithmetic sequence are: Let us verify this pattern for the above example.Ī, a + d, a + 2d, a + 3d, a + 4d. Thus, an arithmetic sequence can be written as a, a + d, a + 2d, a + 3d. is an arithmetic sequence because every term is obtained by adding a constant number (3) to its previous term. The following is an arithmetic sequence as every term is obtained by adding a fixed number 4 to its previous term.Ĭonsider the sequence 3, 6, 9, 12, 15. It is a "sequence where the differences between every two successive terms are the same" (or) In an arithmetic sequence, "every term is obtained by adding a fixed number (positive or negative or zero) to its previous term". 1.ĭifference Between Arithmetic Sequence and Geometric SequenceĪn arithmetic sequence is defined in two ways. Let us learn the definition of an arithmetic sequence and arithmetic sequence formulas along with derivations and a lot more examples for a better understanding. If we want to find any term in the arithmetic sequence then we can use the arithmetic sequence formula. The formula to find the sum of first n terms of an arithmetic sequence.The formula for finding n th term of an arithmetic sequence.We have two arithmetic sequence formulas. For example, the sequence 1, 6, 11, 16, … is an arithmetic sequence because there is a pattern where each number is obtained by adding 5 to its previous term. A sequence is a collection of numbers that follow a pattern. Therefore, all leaf nodes - the echo() calls - would be visited left-to-right, regardless of the precedence of operators joining them.The arithmetic sequence is the sequence where the common difference remains constant between any two successive terms. After the left operand has been evaluated, the right operand is evaluated in the same fashion. The left operand of this operator is first evaluated, which may be composed of higher-precedence operators (such as a call expression echo("left", 4)). Evaluation starts from the outermost group - which is the operator with the lowest precedence ( / in this case). Īfter all operators have been properly grouped, the binary operators would form a binary tree. If you are familiar with binary trees, think about it as a post-order traversal. log ( echo ( "left", 4 ) / echo ( "middle", 3 ) ** echo ( "right", 2 ) ) // Evaluating the left side // Evaluating the middle side // Evaluating the right side // 0.4444444444444444 log ( echo ( "left", 4 ) ** echo ( "middle", 3 ) ** echo ( "right", 2 ) ) // Evaluating the left side // Evaluating the middle side // Evaluating the right side // 262144 // Exponentiation operator (**) has higher precedence than division (/), // but evaluation always starts with the left operandĬonsole. Unicode character class escape: \p // Exponentiation operator (**) is right-associative, // but all call expressions (echo()), which have higher precedence, // will be evaluated before ** doesĬonsole.Character class escape: \d, \D, \w, \W, \s, \S.Enumerability and ownership of properties.


 0 kommentar(er)
0 kommentar(er)
